Significant figures

Ducksters.com
Calculations involving Significant figures-
Rule 1-
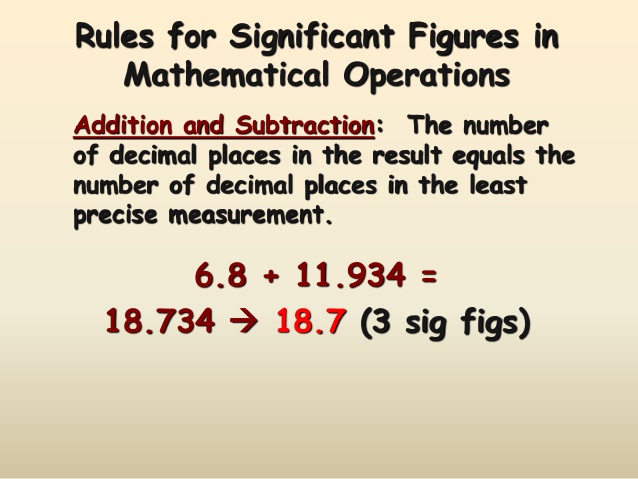
When addition & subtraction of digits is carried out, the result should be reported to the same number of decimal places as that of the term with the least number of decimal places.
Ex 1) Calculate the results of the following measurements to the appropriate number of significant figures.
2.1+ 5.32+ 6.007
Solution – Add the digits,
2.1+ 5.32+ 6.007= 13.427
The least precise value is 2.1, it has only one digit after the decimal point. The sum is 13.427.The reported sum should be only up to one digit after the decimal. Thus answer is 13.4
Ex 2) Calculate the results of the following measurements to the appropriate number of significant figures.
6.21+ 12.314+ 0.0073
Solution – Add the digits,
6.21+ 12.314+ 0.0073= 18.5313
The least precise value is 6.21, it has two-digit after the decimal point. The sum is 18.5313. The reported sum should be up to two-digit after the decimal. Thus answer is 18.53
Ex 3) Calculate the results of the following measurements to the appropriate number of significant figures.
2.1+ 74.632
Solution – Add the digits,
2.1+ 74.632= 76.732
The least precise value is 2.1, it has only one digit after the decimal point. The sum is 76.732. The reported sum should be only up to one digit after the decimal. Thus answer is 76.7
Ex 4) Calculate the results of the following measurements to the appropriate number of significant figures.
45.3245-24.14
Solution – subtract the digits,
45.3244-24.14= 21.1844
The least precise value is 24.14, it has two-digit after the decimal point. The subtracted value is 21.1844,The correct answer should be up to two-digit after the decimal. Thus the correct answer is 21.18
Rule 2-
When multiplication & division of digits is carried out, the result should be reported to the same number of decimal places as that of the term with the least number of decimal places. or as the least precise term used in the calculation.
Ex 1) Calculate the results of the following measurements to the appropriate number of significant figures.
0.67 x 3.124
Solution – Multiply the digits,
0.67 x 3.124= 2.09308
The least precise value is 0.67, it has two-digit after the decimal point. The result of the multiplication is 2.09308, The correct answer should be up to two-digit after the decimal. Thus the correct answer is 2.09
Ex 2) Calculate the results of the following measurements to the appropriate number of significant figures.
6.7 x 312.2/ 0.435 x 2345.4
Solution – Multiply the digits,
6.7 x 312.2/ 0.435 x 2345.4= 2.0502
The least precise value in both numerator & denominator is one after the decimal point. The correct answer should be only up to one digit after the decimal. Thus the correct answer is 2.05
Ex 3) What is the difference between 3.0 gm, 3.00 gm & 3.000 gm?
Solution- The value 3.0 has two significant figures, while 3.00 & 3.000 has 3 & 4 significant figures respectively. This indicates that the third measurement (i-e 3.000) is more precise.
3.0 gm indicates the mass is between 2.9 & 3.1 gm, it means mass is 3.0+/-0.1 gm.
3.00 gm indicates the mass is between 2.99 & 3.01 gm, it means mass is 3.00+/-0.01 gm.
3.000 gm indicates the mass is between 2.999 & 3.001 gm, it means mass is 3.000+/-0.001 gm.