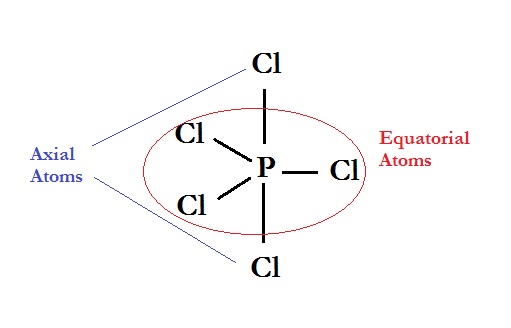
Dissociation of phosphorous penta chloride
source : slide player.net
Dissociation of phosphorous penta chloride
Dissociation of PCl5 takes place accprding to the eqn
PCl5 
PCl3 +Cl2
Let ‘a’ moles of phosphorous penta chloride be taken in a closed vessel of Volume ‘V’ litre. It is heated till equilibrium is established, x moles of PCl5 are dissociated into PCl3 & Cl2 . Therefore x moles of PCl5 give x moles of PCl3 & x moles of Cl2.
PCl5 PCl3 + Cl2
Initial Conc. (mole/l) a/V O O
Concn at eq. (Mol/l) ( a-x) /V x/V x/V
Applying law of mass action,
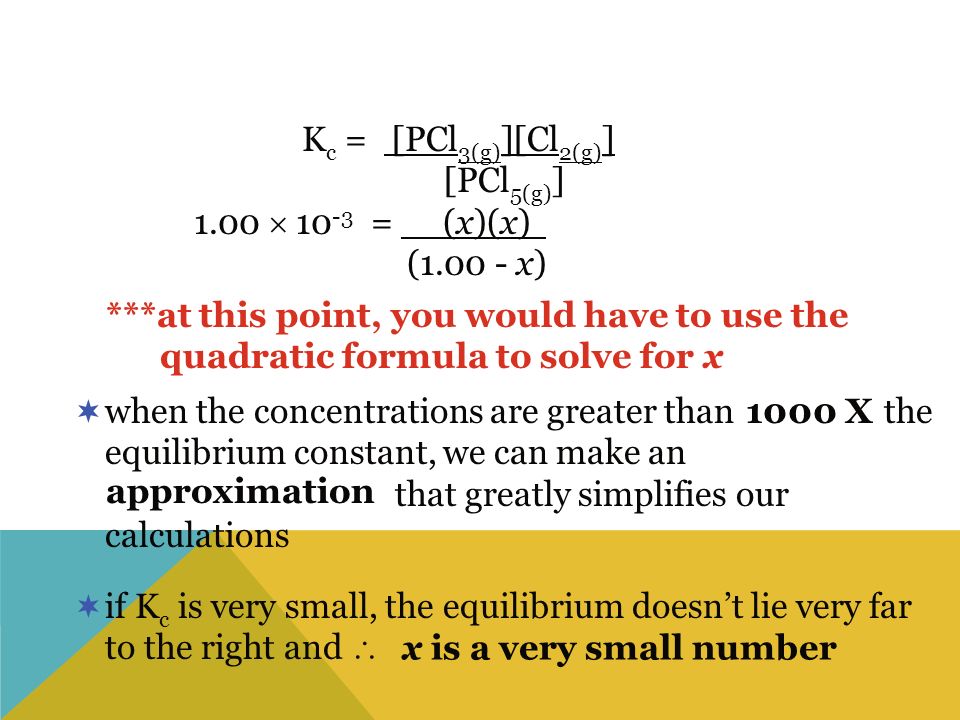
Kc =[ PCl3 ] [ Cl2 ] /[ PCl5 ]
Kc =[ ( x/V) ( x/V)]/[ ( a-x)/V]
Kc =x2 / ( a-x) V
Factors affecting Equilibrium constant-
(i) Effect of Pressure
When pressure is increased, the volume is decreased Hence equilibrium will shift in backward direction. For Kc to remain constt. x will decrease. Hence dissociation of phosphorous penta chloride decreases & equilibrium will shift in the backward direction.
If pressure is decreased, the equilibrium will shift in the forward direction & dissociation of PCl5 increases.
(ii) Effect of Temp.
PCl5 PCl3 +Cl2 – Qk. Cal.
Dissociation of PCl5 is an endothermic reacn. For endothermic reaction, increase in temp. increases the value of equi. constt. & the equilibrium is shifted in the forward direction.
(iii) Effect of Concn–
If concn of any of the reactants or products is changed , the equilibrium is disturbed but Kc remains constt.
If the concn of PCl5 is increased then concentration of PCl3 & Cl2 also increase. Then equilibrium will shift in forward direction & dissociation of PCl5 increases..
(iv) Effect of inert gas– At constt volume no effect of adding inert gas. At constt pressure volume increases on adding inert gas therfore eqilibrium will shift in forward direction
Q- 3 moles of PCl5 is heated in a flask of 4 litre volume. At equilibrium it dissociates to give 40% PCl3 & Cl2 . Calculate Kc.
Soln Amount of dissociation ‘ x ‘= 3 x 40 /100 =1.2 mole
V= 4 litre
PCl5 PCl3 + Cl2
Initial Conc. (mole/l) 3 /V 0 0
Concn at eq. (Mol/l) 3-1.2 /V 1.2 /V 1.2/V =1.8/V
Kc =[ PCl3 ] [ Cl2 ] /[ PCl5 ]
Kc =[ ( 1.2/V) ( 1.2/V)]/[1.8/V]
=( 1.2 x 1.2)/( 1.8 x 4)
Kc =0.2
- 0.34 moles of PCl5 is heated in a closed vessel of 1 litre capacity at 500 K. At equilibrium 0.1 moles of PCl5 is dissociated. calculate Kc.
Ans. v=1 litre
PCl5 PCl3 + Cl2
Initial Conc. (mole/l) 0.34/V O O
Concn at eq. (Mol/l) 0.34-0.1 /V 0.1/V 0.1 /V =0.24/V
Kc =[ PCl3 ] [ Cl2 ] /[ PCl5 ]
Kc =[ ( 0.1/V) ( 0.1/V)]/[ 0.24/V]
=(0.1 x 0.1)/(0.24 x 1)
=0.042 Ans
Q –625.5 gm. PCl5 is heated in a vessel of 5 litre capacity at equilibrium 45% of PCl5 is dissociated into PCl3 & Cl2. calculate Kc.=?
Ans. m of PCl5 = 31+5+35.5
=208.5
Initial Concn of PCl5 = 625.5/208.5 =3
Amt. of dissociation of PCl5 =(3 x 45)/100
=1.35 mole
PCl5 PCl3 + Cl2
Initial Conc. (mole/l) 3 /V O O
Concn at eq. (Mol/l) 3-1.35/V 1.35 /V 1.35 /V =1.65/V
Kc =[ PCl3 ] [ Cl2 ] /[ PCl5 ]
Kc =[ ( 1.35/V) ( 1.35/V)]/[ ( 1.65)/V]
=(1.35 x 1.35)/(1.65 x 5)
Kc =0.22 Ans
Relation between degree of dissociation & Vapour density-
Suppose degree of dissociation at equilibrium is x
PCl5 PCl3 + Cl2
Initial no. of moles 1 O O
mole at equi 1-x x x
Initial no. of moles = 1+0+0=1
Total moles at Equilibrium = 1-x+x+x= 1+x
‘D’ is the vapour density when there is no dissociation
‘d’ is the observed vapour density at a temp. when degree of dissociation is x
No.of moles ∝ 1/vapour density
total moles at equilibrium /initial moles =D/d
(1+x) /1 =D/d
x = (D-d) /d
- The V. density of PCl5 at 600K is 61 . Calculate the degree of dissociation of PCl5 at this temp. Mol wt. of PCl5= 208.5
Ans. d=61
D =mol,wt /2 =208.5/2 =104.25
(1+x) /1 =D/d
( 1+x) /1 = 104.25 /61
x =0.709
% of x =70.9 % Ans.
